EXPERIMENT
RESPONSE
OF SECOND ORDER SYSTEMS (Step Change)
1. OBJECTIVE
To study the response of second order system
subjected to step change.
2. INTRODUCTION
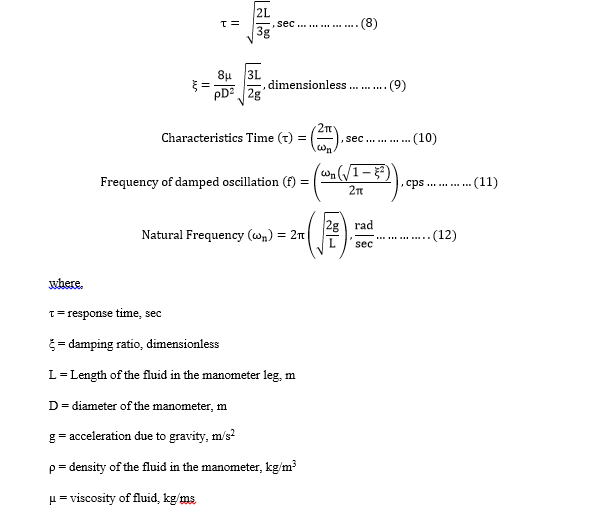
Second order response
3. EXPERIMENTAL SET-UP
 |
| Experimental Setup of second order system |
The
above apparatus used to study the response of second order system subjected to
step change. Two
U tube manometer with water and mercury inside them are used. The inside
diameter of the tube which is filled with mercury is 5 mm and tube which is
filled with water have 22mm inside diameter. Two
valves are provided to control the amount of change. The length of the fluid column in
the manometer is L. At time t = 0, a pressure difference is imposed across the
legs of the manometer and impulsively released.
4.
PROCEDURE
1. The
mercury should be at equal lengths on both the arms
2. The
vent connection is closed by putting a finger on it
3. The
needle valve and the vent are adjusted to raise the mercury to 200mm or above
from the original level
4. Note
the mercury level and remove the finger from the vent and start recording the
oscillations
5. The
process is repeated for different values of step change
6. Repeat
steps 1-5 for water manometer
5. RESULTS
 |
| Plots for theoretical and Experimental data for mercury manometer |
Above Figures shows the plot between
the manometer responses with respect to time. It can be observed that the
theoretical and experimental values are different and reach to their steady
state conditions are different response times. System is underdamped with
damping coefficient <1. Characteristics Time,
Natural Frequency, Frequency of Damped Oscillations are 0.197, 31.924 and 5.080 respectively. Decay Ratio, Overshoot
and period of oscillation for mercury manometer are 0.911, 0.954 and 0.197 respectively.
 |
| Plots for theoretical and Experimental data for water manometer |
Above Figures shows the plot between
the manometer responses with respect to time. It can be observed that the
theoretical and experimental values are almost same and reach to their steady
state conditions are different response times. System is underdamped with
damping coefficient <1. For mercury
manometer, Characteristics Time, Natural Frequency, Frequency of Damped
Oscillations are 0.231, 27.160 and 4.343 respectively. Decay Ratio, Overshoot and period of oscillation
for mercury manometer are 0.953, 0.976 and 0.231 respectively for mercury
manometer.
6.
CONCLUSION
The aim of this experiment was to
study the response of a second order system. The system under consideration was
a mercury manometer and a water manometer. . System is underdamped with damping
coefficient <1. Characteristics Time,
Natural Frequency, Frequency of Damped Oscillations are 0.197, 31.924 and 5.080 respectively. Decay Ratio, Overshoot
and period of oscillation for mercury manometer are 0.911, 0.954 and 0.197 respectively. For water manometer, Characteristics Time,
Natural Frequency, Frequency of Damped Oscillations are 0.231, 27.160 and
4.343 respectively. Decay Ratio, Overshoot and period of oscillation for mercury
manometer are 0.953, 0.976 and 0.231 respectively for water
manometer.
7. REFERENCES
[1]
|
D. R. Coughanowr and S. E. LeBlanc, "Higher-Order
Systems : Second Order and Transportation Lag," in Process System Analysis and Control,
New York, McGraw Hills, 2009, p. 137.
|
[2]
|
D. R. Coughanowr
and S. E. LeBlanc, "Higher-Order Systems: Second Order and
Transportation Lag," in Process
System Analysis and Control, New York, McGray Hills, 2009, p. 141.
|
[3]
|
D. R. Coughanowr
and S. E. LeBlanc, "HIgher-Order Systems: Second Order and
Transportation Lag," in Process
System Analysis and Control, New York, McGraw Hills, 2009, pp.
142-144.
|